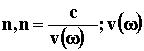 is the group velocity of light in
the material and c is the velocity of light in vacuum.
is the group velocity of light in
the material and c is the velocity of light in vacuum.CERENKOV EFFECT
The
Cerenkov electromagnetic radiation, usually bluish light, is emitted by a beam
of high-energy charged particles passing through a transparent medium at a speed
greater than the speed of light in that medium.
The
effect is similar to that of a sonic boom when an object moves faster than the
speed of sound; in this case the radiation is a shock wave set up in the
electromagnetic field.
In
fig.1, a charged particle moves with speed
![]() , where
, where
![]() through a depressive medium with
the refraction index,
through a depressive medium with
the refraction index,
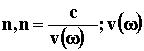 is the group velocity of light in
the material and c is the velocity of light in vacuum.
is the group velocity of light in
the material and c is the velocity of light in vacuum.
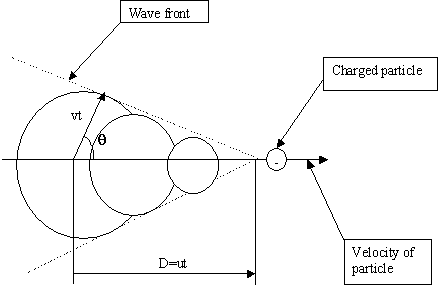
Fig.1 Cerenkov effect
The
particle traveling with speed
![]() , will emit photons contained in a cone, at an angle q with respect to its direction, where qcan be computed
with relation:
, will emit photons contained in a cone, at an angle q with respect to its direction, where qcan be computed
with relation:
![]() (2.1)
(2.1)
or
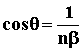 , where
, where
![]() (2.2)
(2.2)
If
we compute the speed of light in a depressive medium, such as water for which
n=1,3, we get:
![]() (2.3)
(2.3)
so
the particles must have a velocity higher than this value.
For
beta particles with the rest mass
![]() , one can calculate the minimum value of the total energy at which Cerenkov
radiation is emitted, using the following formula:
, one can calculate the minimum value of the total energy at which Cerenkov
radiation is emitted, using the following formula:
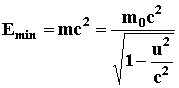 (2.4)
(2.4)
Computing
(4) for
![]() gives
gives
![]()
The
Cerenkov photoemission may be studied as a relativistic effect in which the
conservation of energy and linear momentum must both be satisfied.
In
Fig.2, there is represented a charged particle with initial momentum
![]() , rest mass m0 and velocity
, rest mass m0 and velocity
![]() , moving through a medium with refraction index n, faster than light in the same
medium. A photon with frequency n
or wavelength l and momentum
, moving through a medium with refraction index n, faster than light in the same
medium. A photon with frequency n
or wavelength l and momentum
![]() occurs. The charged particle travels with the final momentum
occurs. The charged particle travels with the final momentum
![]() , after the photon emission.
, after the photon emission.
One
can write:
![]()

Fig.2
Conservation
of linear momentum gives:
![]() (2.5)
(2.5)
and
![]() (2.6)
(2.6)
Conservation
of total energy gives:
![]() (2.7)
(2.7)
where
![]() is the photon energy.
is the photon energy.
The
fundamental relation between the total energy and momentum gives:
![]() and
and
![]() (2.8)
(2.8)
Plugging
(2.8) into (2.7) gives:
![]()
or
![]()
![]() (2.9)
(2.9)
and
![]() (2.10)
(2.10)
where
the final momentum p is unknown.
This
one can be eliminated by using (2.6) and (2.10)
![]() (2.11)
(2.11)
The
photon momentum
![]() can be written:
can be written:
![]() (2.12)
(2.12)
where
the wavelength is expressed as:
![]() (2.13)
(2.13)
Replacing
(2.13) into (2.11) gives:
![]() (2.14)
(2.14)
Eq.
(2.14) becomes:
![]() (2.15)
(2.15)
where
the particle energy Ei is:
 (2.16)
(2.16)
Therefore:
 (2.17)
(2.17)
so
 (2.18)
(2.18)
Calculating
![]() from (2.4) and (2.8) and knowing n
from the blue light, one can estimate the last term in (2.18) which is very
small and can be neglected.
from (2.4) and (2.8) and knowing n
from the blue light, one can estimate the last term in (2.18) which is very
small and can be neglected.
Therefore:
![]() (2.19)
(2.19)
This
effect was used for building up the Cerenkov counters for detecting and counting
high-energy charged particles. A photo multiplier tube registers the resulted
radiation.
According
to the CPT theorem, one can exchange the places of particles in the Cerenkov
process (
![]() ). Through the charge conjugation, we get:
). Through the charge conjugation, we get:
![]()
where
![]() and
and
![]() are a pair of particles both an
electron and positron obtained from a single gamma ray:
are a pair of particles both an
electron and positron obtained from a single gamma ray:
Thus,
the antiparticle generation is equivalent with the particle annihilation.
The
conservation laws (energy and linear momentum) are satisfied in the pair
production, and allow us to determine the momentum, direction and magnitude of
the gamma ray.
In
fig.3
![]() are momenta of gamma-ray photon,
electron and positron.
are momenta of gamma-ray photon,
electron and positron.
Having
the same rest masses, one can assume that resulted particles will have
![]() and
and
![]() , so:
, so:

 (2.20)
(2.20)
where
E1 and E2 are total energies of the resulted particles.
 (2.21)
(2.21)
where
![]()
Using
the Cerenkov radiation to measure the trajectory of the pairs, we can determine
the momentum of the gamma-ray.